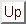) and (
) and ( )
the rms slope error of the optical aberration
)
the rms slope error of the optical aberration 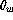 is
given by the expression
is
given by the expression



On the 8-m primary mirror of the VLT, wind will have both a negative effect as it will deform dynamically the surface shape and a positive effect as it will decrease the mirror seeing when the mirror is warmer than ambient air. To analyze this combination we need to parameterize both effects in the same terms.
Dynamic deformations due to wind buffeting
Combining equations ( ) and (
) and ( )
the rms slope error of the optical aberration
)
the rms slope error of the optical aberration 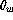 is
given by the expression
is
given by the expression
where U is the mean wind flow speed about 60 cm above the mirror.
Inputing the data of the VLT 8.2-m mirror
(see page  )
at the pressure height of
)
at the pressure height of  2500 m (
2500 m (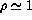 )
equation (6.1) becomes
)
equation (6.1) becomes
Mirror seeing
The mirror
seeing FWHM angle 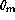 is parameterized from equations
(
is parameterized from equations
( ) and (
) and ( ) as
) as
obtaining for the particular case of the VLT mirror and an average
temperature 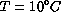

Figure 6.3: Total image quality 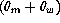 of the VLT
8-m primary mirror as a function of the wind
speed measured 60 cm above the mirror surface
of the VLT
8-m primary mirror as a function of the wind
speed measured 60 cm above the mirror surface
For small speed values of (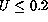 m/s),
approaching the free convection condition,
m/s),
approaching the free convection condition,
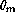 is parameterized by equation (
is parameterized by equation ( ):
):

The quadratic sum of 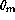 and
and
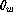 are plotted in fig. 6.3 for different
positive mirror-air temperature differences.
are plotted in fig. 6.3 for different
positive mirror-air temperature differences.