


Next: The microstructure of
Up: Thermal turbulencelight
Previous: Thermal turbulencelight
[Tatarskii]
describes the microstructure of the temperature field in a fluid
with homogeneous and isotropic turbulence, characterized in particular by its
dissipation rate of kinetic energy  , similarly to Kolmogorov's
analysis of the turbulent velocity field. The analysis leads to the
definition of an inner scale corresponding to the smallest
temperature variations, which is determined by the thermal diffusivity
, similarly to Kolmogorov's
analysis of the turbulent velocity field. The analysis leads to the
definition of an inner scale corresponding to the smallest
temperature variations, which is determined by the thermal diffusivity
 and the dissipation rate
and the dissipation rate  of momentum:
of momentum:
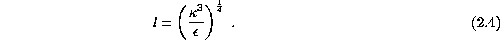
Continuing the analogy with the velocity field,
Tatarskii finds then an inertial domain, between
the outer scale of turbulence L and l
where the temperature structure function
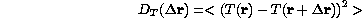
has the form:

where 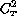 is the temperature structure coefficient.
is the temperature structure coefficient.
 characterizes completely the local thermal turbulence at
a give time, has units (K
characterizes completely the local thermal turbulence at
a give time, has units (K m
m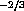 ) and is therefore formally
defined as:
) and is therefore formally
defined as:
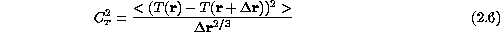
for a separation 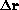 in the inertial domain.
in the inertial domain.
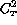 is also related to the one-dimensional temperature spectrum
which in the inertial domain has the form:
is also related to the one-dimensional temperature spectrum
which in the inertial domain has the form:
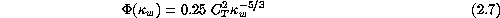
where 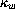 is the streamwise component of wavenumber.
is the streamwise component of wavenumber.
Moreover, [Tatarskii] notes that in the inertial domain
 should be a function of only
should be a function of only  ,
,
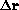 and the temperature dissipation rate
and the temperature dissipation rate
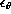 .
Dimensional reasoning leads then to
.
Dimensional reasoning leads then to

where 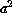 is a constant found to be equal to about 3.
is a constant found to be equal to about 3.
The value of 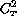 at a given point can be measured by special
temperature sensors. Sometimes two sensors are used, with a separation
at a given point can be measured by special
temperature sensors. Sometimes two sensors are used, with a separation
 of the order or 1 meter, but often the measurement is
taken with one sensor only. The data are then processed assuming
the Taylor hypothesis of "frozen turbulence" in which time and
spatial intervals are linked by the mean wind speed as:
of the order or 1 meter, but often the measurement is
taken with one sensor only. The data are then processed assuming
the Taylor hypothesis of "frozen turbulence" in which time and
spatial intervals are linked by the mean wind speed as:

This hypothesis implies that velocity fluctuations are small with
respect to the mean value, which is generally the case with not
exceedingly gusty winds with a mean speed of at least 4 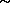 5 m/s.
The temperature
structure coefficient can then be evaluated from the variations of
temperature at a single point as:
5 m/s.
The temperature
structure coefficient can then be evaluated from the variations of
temperature at a single point as:
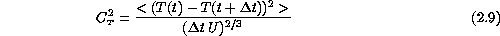
The temperature sensors for the measurement of 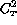 must have
a high resolution and a large dynamic range.
must have
a high resolution and a large dynamic range. 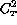 may vary from
may vary from
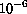 K
K m
m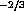 during the night on an excellent site to
during the night on an excellent site to
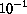 K
K m
m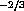 during the day and convection from the ground.
The required bandwidth is determined by the -5/3 slope of the
temperature
spectrum (
during the day and convection from the ground.
The required bandwidth is determined by the -5/3 slope of the
temperature
spectrum ( ) and will generally be at least 100 or
200 Hz.
) and will generally be at least 100 or
200 Hz.
Direct measurements of 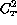 from a fixed setup are of course possible
only close to the ground while one-time vertical profiles over a larger
height can be obtained by aerostatic balloons. The already mentioned
SODAR can measure refraction turbulent profiles in the boundary layer
with a height range between 50 and 800 m.
The profiles of
from a fixed setup are of course possible
only close to the ground while one-time vertical profiles over a larger
height can be obtained by aerostatic balloons. The already mentioned
SODAR can measure refraction turbulent profiles in the boundary layer
with a height range between 50 and 800 m.
The profiles of 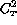 in the high atmosphere and
in the boundary layer have been also the
objects of many studies aiming at determining their relationship
with height and the atmosphere parameters: see in particular
[Coulman 86], [Coulman 88] and [Consortini].
in the high atmosphere and
in the boundary layer have been also the
objects of many studies aiming at determining their relationship
with height and the atmosphere parameters: see in particular
[Coulman 86], [Coulman 88] and [Consortini].



Next: The microstructure of
Up: Thermal turbulencelight
Previous: Thermal turbulencelight
Lorenzo Zago, lorenzo.zago@heig-vd.ch,
Mon Nov 6 23:33:14 GMT+0100 1995
 , similarly to Kolmogorov's
analysis of the turbulent velocity field. The analysis leads to the
definition of an inner scale corresponding to the smallest
temperature variations, which is determined by the thermal diffusivity
, similarly to Kolmogorov's
analysis of the turbulent velocity field. The analysis leads to the
definition of an inner scale corresponding to the smallest
temperature variations, which is determined by the thermal diffusivity
 and the dissipation rate
and the dissipation rate  of momentum:
of momentum:



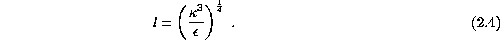
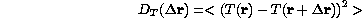

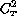 is the temperature structure coefficient.
is the temperature structure coefficient.  characterizes completely the local thermal turbulence at
a give time, has units (K
characterizes completely the local thermal turbulence at
a give time, has units (K m
m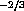 ) and is therefore formally
defined as:
) and is therefore formally
defined as:
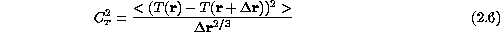
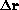 in the inertial domain.
in the inertial domain.
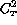 is also related to the one-dimensional temperature spectrum
which in the inertial domain has the form:
is also related to the one-dimensional temperature spectrum
which in the inertial domain has the form:
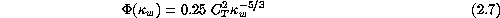
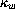 is the streamwise component of wavenumber.
is the streamwise component of wavenumber. should be a function of only
should be a function of only  ,
,
 and the temperature dissipation rate
and the temperature dissipation rate
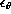 .
Dimensional reasoning leads then to
.
Dimensional reasoning leads then to

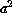 is a constant found to be equal to about 3.
is a constant found to be equal to about 3.
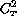 at a given point can be measured by special
temperature sensors. Sometimes two sensors are used, with a separation
at a given point can be measured by special
temperature sensors. Sometimes two sensors are used, with a separation
 of the order or 1 meter, but often the measurement is
taken with one sensor only. The data are then processed assuming
the Taylor hypothesis of "frozen turbulence" in which time and
spatial intervals are linked by the mean wind speed as:
of the order or 1 meter, but often the measurement is
taken with one sensor only. The data are then processed assuming
the Taylor hypothesis of "frozen turbulence" in which time and
spatial intervals are linked by the mean wind speed as:

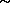 5 m/s.
The temperature
structure coefficient can then be evaluated from the variations of
temperature at a single point as:
5 m/s.
The temperature
structure coefficient can then be evaluated from the variations of
temperature at a single point as:
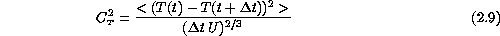
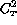 must have
a high resolution and a large dynamic range.
must have
a high resolution and a large dynamic range. 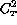 may vary from
may vary from
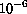 K
K m
m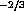 during the night on an excellent site to
during the night on an excellent site to
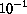 K
K m
m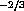 during the day and convection from the ground.
The required bandwidth is determined by the -5/3 slope of the
temperature
spectrum (
during the day and convection from the ground.
The required bandwidth is determined by the -5/3 slope of the
temperature
spectrum (
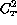 from a fixed setup are of course possible
only close to the ground while one-time vertical profiles over a larger
height can be obtained by aerostatic balloons. The already mentioned
SODAR can measure refraction turbulent profiles in the boundary layer
with a height range between 50 and 800 m.
The profiles of
from a fixed setup are of course possible
only close to the ground while one-time vertical profiles over a larger
height can be obtained by aerostatic balloons. The already mentioned
SODAR can measure refraction turbulent profiles in the boundary layer
with a height range between 50 and 800 m.
The profiles of 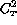 in the high atmosphere and
in the boundary layer have been also the
objects of many studies aiming at determining their relationship
with height and the atmosphere parameters: see in particular
[
in the high atmosphere and
in the boundary layer have been also the
objects of many studies aiming at determining their relationship
with height and the atmosphere parameters: see in particular
[